Page 98 - Kỷ yếu hội thảo khoa học lần thứ 12 - Công nghệ thông tin và Ứng dụng trong các lĩnh vực (CITA 2023)

82

[14]. In [14], the authors reported that the arithmetic mean and the median lead to

to the other. The geometric mean turns out to be farther away from BBV in almost all
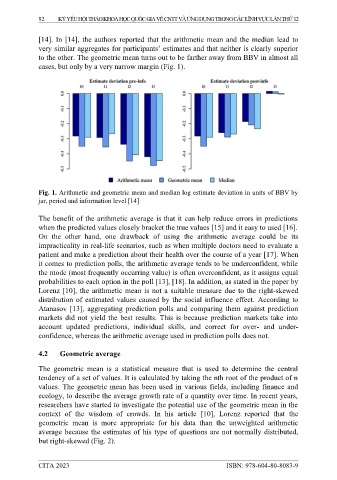
cases, but only by a very narrow margin (Fig. 1).

Fig. 1. Arithmetic and geometric mean and median log estimate deviation in units of BBV by
jar, period and information level [14]

The benefit of the arithmetic average is that it can help reduce errors in predictions
when the predicted values closely bracket the true values [15] and it easy to used [16].
On the other hand, one drawback of using the arithmetic average could be its
impracticality in real-life scenarios, such as when multiple doctors need to evaluate a
patient and make a prediction about their health over the course of a year [17]. When
it comes to prediction polls, the arithmetic average tends to be underconfident, while
the mode (most frequently occurring value) is often overconfident, as it assigns equal
probabilities to each option in the poll [13], [18]. In addition, as stated in the paper by
Lorenz [10], the arithmetic mean is not a suitable measure due to the right-skewed
distribution of estimated values caused by the social influence effect. According to
Atanasov [13], aggregating prediction polls and comparing them against prediction
markets did not yield the best results. This is because prediction markets take into
account updated predictions, individual skills, and correct for over- and under-
confidence, whereas the arithmetic average used in prediction polls does not.

4.2 Geometric average

The geometric mean is a statistical measure that is used to determine the central
tendency of a set of values. It is calculated by taking the nth root of the product of n
values. The geometric mean has been used in various fields, including finance and
ecology, to describe the average growth rate of a quantity over time. In recent years,
researchers have started to investigate the potential use of the geometric mean in the
context of the wisdom of crowds. In his article [10], Lorenz reported that the
geometric mean is more appropriate for his data than the unweighted arithmetic
average because the estimates of his type of questions are not normally distributed,
but right-skewed (Fig. 2).

CITA 2023 ISBN: 978-604-80-8083-9